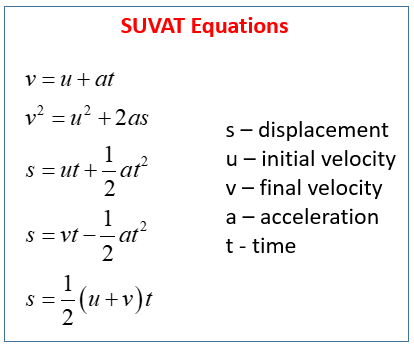
If you’ve ever wondered what the Suvat equations are, you’ve come to the right place. They’re a single, first-order non-linear PDE that can be used to work out initial and final speed, displacement, acceleration, and time. They’re also integral concerning a more general law. This article will walk you through the basics of Suvat equations.
Suvat equations are a single first order non-linear PDE
The Suvat equations are a single unitary first-order non-linear PDE, and the solution to this model depends on two arbitrary constants, T and f. In this model, acceleration is constant. Thus, the total distance is f(C1) x T. Students should ensure that their measurements are consistent and use the same SI unit in all of their calculations.
The Suvat equations are a single, first-order non-linear PDE with a large symmetry group. All solutions are found in the tangent space to a particular point, and the resulting solution space is a single, finite-dimensional compact manifold. The Navier-Stokes equation is a similar example, with a large symmetry group.
They work out initial and final speed, time, displacement, and acceleration.
The SUVAT equations are used to calculate an object’s initial and final speed, time, and displacement. The equations are often called the “SUV equations” as they are commonly used to calculate an object’s initial and final speed. Often the initial and final rate is known, but the acceleration of a body is not. Therefore, the SUVAT equations can only be used to determine the speed of an object moving in a straight line.
The Suvat equations can be compared with the kinematics of motion. A constant acceleration (CA) calculates the initial and final speed of a body moving under gravity. The equations have four variables, three of which are required for the calculation. The initial position vector, initial velocity, and initial time are used to calculate the final speed and displacement.
They are integral concerning
Look no further if you are looking for a calculator for the SUVAT equation. You can get the answer using an online calculator, but that won’t do you good if you are stuck in the middle of a question. This can be frustrating, especially since you’ve been waiting 10 minutes to load the page and are trying to answer an important question. The problem is that you have just finished learning the SUVAT equation ages ago and are about to move onto friction and normal force. You’re probably in the middle of a statistics class.
SUVAT equations involve five variables: initial velocity, final velocity, acceleration, and time is taken. These five variables describe a system in motion with uniform acceleration. The final rate, v, is the speed of an object. The time taken to reach that velocity is t. This equation is the one used to define the SUVAT. It arbitrarily connects the variables.
They are integral to more general law
The SUVAT equations describe the relationship between an object’s initial and final velocity. The equations are an integral part of a more general law of motion called kinematics. These equations are usually derived from the definitions of the kinematic quantities, such as displacement, initial and final velocity, acceleration, and time taken. The equations for four variables are known as the kinematic equations.
The first SUVAT equation relates the acceleration of an object with time. The second SUVAT equation relates the acceleration and the total distance traveled. The average speed of an object over a time interval is the average speed. However, the instantaneous rate of an object at a given point may be different. When applying the SUVAT equations, it is essential to note that the vertical velocity is zero before impact and a significant upward acceleration occurs immediately after effects.
They are integral to a Lorentz force
A Lorentz force is an electric or magnetic force applied to charged particles in motion. The force can be described as a non-linear function of time. The Suvat equations are integrals of a Lorentz force. These equations are often called SUVAT equations. Their solution is derived from kinematic quantities, such as the velocity of a particle, its energy, and its momentum.
There are several ways to solve the Suvat equations. Firstly, consider that the first equation is an integral of a Lorentz force. If there is one equation for the position of a point charge, it must be a quadratic one. The third equation describes the position vector and is a quadratic one. It contains the initial velocity vector and position vector.
They are integral to a kinematic equation
The four variables of the Suvat equation are initial velocity, position, and acceleration. If a body is moving under gravity, it experiences constant acceleration. The other two variables can be calculated by using the three variables. The equations contain four variables, but the last two are a particular case. Hence, the Suvat equations are integral to a kinematic equation.
The SUVAT equations only apply when the initial and final velocities are constant. Unless the initial and final rates are stable, you’ll need to use calculus to find the average speeds. However, this is easier said than done. For most practical situations, like the orbiting of planets or accelerating a motor car, the SUVAT equations are sufficient.